Trigonométrie : Exercice 13 2ème année secondaire

Calcul dans IRProblèmes du 1er et du second degréNotion de polynômesArithmetiqueCalcul vectorielBarycentreTranslationsHomothetiesRotationsSuitesFonctionsTrigonométrieGéométrie analytiqueGéométrie dans l'espaceStatistiquesQCM

24 exercices

Exercice 13 --- (id : 817)
Trigonométrie: Exercice 13
correction
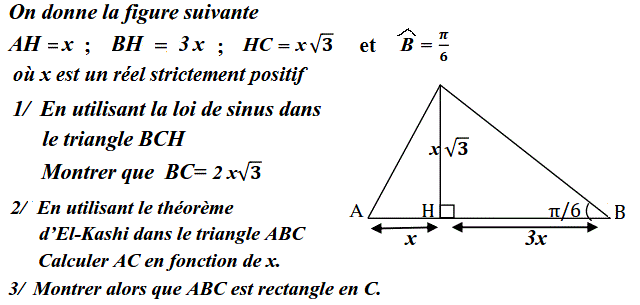
1 Dans le triangle BCHBCH on a : sin⁡B^CH=sin⁡C^BH=sin⁡H^BC\dfrac{\sin \widehat{B}}{CH}=\dfrac{\sin \widehat{C}}{BH}=\dfrac{\sin \widehat{H}}{BC}
sin⁡B^CH=sin⁡H^BC\dfrac{\sin \widehat{B}}{CH}=\dfrac{\sin \widehat{H}}{BC}
  ⟺  BC=sin⁡H^sin⁡B^.CH\iff BC=\dfrac{\sin \widehat{H}}{\sin \widehat{B}}.CH
  ⟺  BC=sin⁡π2sin⁡π6.x3\iff BC=\dfrac{\sin \frac{\pi}{2}}{\sin \frac{\pi}{6}}.x\sqrt{3}
  ⟺  BC=112.x3=2x3\iff BC=\dfrac{1}{\frac{1}{2}}.x\sqrt{3}=2x\sqrt{3}
2 Dans le triangle ABCABC on a: AC2=BA2+BC2−2.BA.BC.cos⁡B^AC^2=BA^2+BC^2-2.BA.BC.\cos \widehat{B}   ⟺  AC2=(4x)2+(2x3)2−2.4x.2x3.cos⁡π6\iff AC^2=(4x)^2+(2x\sqrt{3})^2-2.4x.2x\sqrt{3}.\cos \dfrac{\pi}{6}   ⟺  AC2=16x2+12x2−16x2332\iff AC^2=16x^2+12x^2-16x^2\sqrt{3}\dfrac{\sqrt{3}}{2}   ⟺  AC2=28x2−24x2=4x2\iff AC^2=28x^2-24x^2=4x^2 donc AC=2xAC=2x
3 BC2+CA2=(2x3)2+(2x)2BC^2+CA^2=(2x\sqrt{3})^2+(2x)^2 =16x2=(4x)2=16x^2=(4x)^2 donc BC2+CA2=BA2BC^2+CA^2=BA^2 d'où ABCABC est un triangle rectangle en CC
<< < 1...4...8910111213 1415161718...23...24 > >>

Charte d'utilisation --- Plan du site
Copyright © 2010-2025 Dhaouadi Nejib - Tunisia