Activités algébriques : Exercice 4 première année secondaire
Activités numériques IActivités numériques IIActivités algébriquesFonctions linéairesEquations et inéquationsFonctions affinesSystème de deux équationsStatistiquesAnglesThalesRapports trigonométriqueVecteurs et translationsActivités dans un repereQuart de tourSections planes solideQCM
77 exercices
Exercice 4 --- (id : 1290)
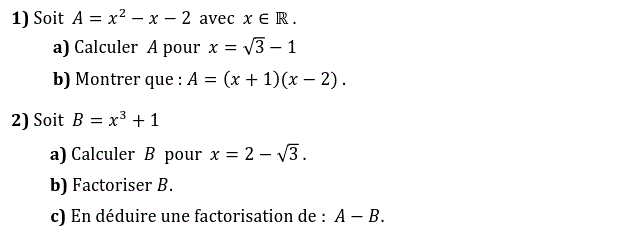
correction
1)
a)
Pour $x=\sqrt{3}-1$ on a:
$$\begin{align*} A&=(\sqrt{3}-1)^2-(\sqrt{3}-1)-2 \\ &=\sqrt{3}^2-2\times \sqrt{3}\times 1+1^2-\sqrt{3}+1-2 \\ &=3-2\sqrt{3}\cancel{+1}-\sqrt{3}\cancel{-1} \\ &=3-3\sqrt{3} \end{align*}$$
$$\begin{align*} A&=(\sqrt{3}-1)^2-(\sqrt{3}-1)-2 \\ &=\sqrt{3}^2-2\times \sqrt{3}\times 1+1^2-\sqrt{3}+1-2 \\ &=3-2\sqrt{3}\cancel{+1}-\sqrt{3}\cancel{-1} \\ &=3-3\sqrt{3} \end{align*}$$
b)
$(x+1)(x-2)$
$=x^2-2x+x-2 $
$=x^2-x-2=A$
$(x+1)(x-2)$
$=x^2-2x+x-2 $
$=x^2-x-2=A$
2)
a)
Pour $x=2-\sqrt{3}$ on a:
$$\begin{align*} B&=(2-\sqrt{3})^3+1 \\ &=(2-\sqrt{3})^2(2-\sqrt{3})+1 \\ &=(4-4\sqrt{3}+3)(2-\sqrt{3})+1 \\ &=(7-4\sqrt{3})(2-\sqrt{3})+1 \\ &=14-7\sqrt{3}-8\sqrt{3}+12+1 \\ &=27-15\sqrt{3} \end{align*}$$
$$\begin{align*} B&=(2-\sqrt{3})^3+1 \\ &=(2-\sqrt{3})^2(2-\sqrt{3})+1 \\ &=(4-4\sqrt{3}+3)(2-\sqrt{3})+1 \\ &=(7-4\sqrt{3})(2-\sqrt{3})+1 \\ &=14-7\sqrt{3}-8\sqrt{3}+12+1 \\ &=27-15\sqrt{3} \end{align*}$$
b)
$B=x^3+1=(x+1)(x^2-x+1)$
c)
$$\begin{align*}
A-B&=(x+1)(x-2)-(x+1)(x^2-x+1) \\
&=(x+1)[(x-2)-(x^2-x+1)] \\
&=(x+1)(x-2-x^2+x-1) \\
&=(x+1)(-x^2+2x-3)
\end{align*}$$