Equations et inéquations : Exercice 18 première année secondaire
Activités numériques IActivités numériques IIActivités algébriquesFonctions linéairesEquations et inéquationsFonctions affinesSystème de deux équationsStatistiquesAnglesThalesRapports trigonométriqueVecteurs et translationsActivités dans un repereQuart de tourSections planes solideQCM
81 exercices
Exercice 18 --- (id : 1584)
correction
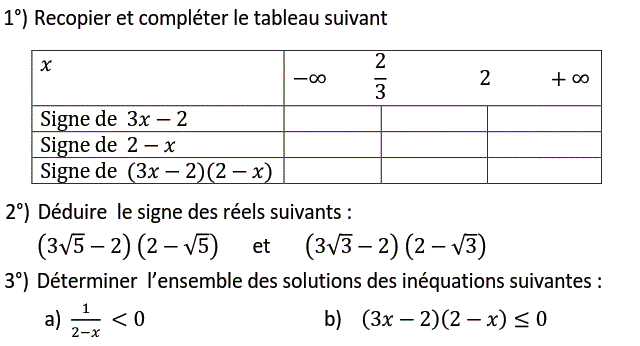
1°)
$$\small
\def\arraystretch{1.2}
\begin{array}{|c|ccccccc|}
\hline
x & -\infty & & \frac{2}{3} & & 2 & & +\infty \\ \hline
3x-2 & & - & 0 & + & & + \\ \hline
2-x & & + & & + & 0 & - \\ \hline
(3x-2)(2-x) & & - & 0 & + & 0 & - \\ \hline
\end{array}$$
2°)
Posons $A(x)=(3x-2)(2-x)$
🔷$\sqrt 5 \in \left]{2;+\infty}\right[$ donc $A(\sqrt 5)<0$ (voir tableau) d'où $(3\sqrt 5-2)(2-\sqrt 5)<0$
🔷$\sqrt 3 \simeq 1,7 \in \left]{\frac{2}{3};2}\right[$ donc $A(\sqrt 3)>0$ d'où $(3\sqrt 3-2)(2-\sqrt 3)>0$
🔷$\sqrt 5 \in \left]{2;+\infty}\right[$ donc $A(\sqrt 5)<0$ (voir tableau) d'où $(3\sqrt 5-2)(2-\sqrt 5)<0$
🔷$\sqrt 3 \simeq 1,7 \in \left]{\frac{2}{3};2}\right[$ donc $A(\sqrt 3)>0$ d'où $(3\sqrt 3-2)(2-\sqrt 3)>0$
3°)
🔷$\dfrac{1}{2-x}<0$ $\iff 2-x<0$ $\iff x\in \left]{2;+\infty}\right[$ (Voir tableau de signe)
Donc $\boxed{S_\Bbb R=\left]{2;+\infty}\right[}$
🔷$A(x)\leqslant 0$ $\iff x\in \left]{-\infty;\frac{2}{3}}\right]\cup \left[{2;+\infty}\right[$ (Voir tableau de signe)
Donc $\boxed{S_\Bbb R=\left]{-\infty;\dfrac{2}{3}}\right]\cup \left[{2;+\infty}\right[}$
Donc $\boxed{S_\Bbb R=\left]{2;+\infty}\right[}$
🔷$A(x)\leqslant 0$ $\iff x\in \left]{-\infty;\frac{2}{3}}\right]\cup \left[{2;+\infty}\right[$ (Voir tableau de signe)
Donc $\boxed{S_\Bbb R=\left]{-\infty;\dfrac{2}{3}}\right]\cup \left[{2;+\infty}\right[}$