Equations et inéquations : Exercice 1 première année secondaire
Activités numériques IActivités numériques IIActivités algébriquesFonctions linéairesEquations et inéquationsFonctions affinesSystème de deux équationsStatistiquesAnglesThalesRapports trigonométriqueVecteurs et translationsActivités dans un repereQuart de tourSections planes solideQCM
81 exercices
Exercice 1 --- (id : 1322)
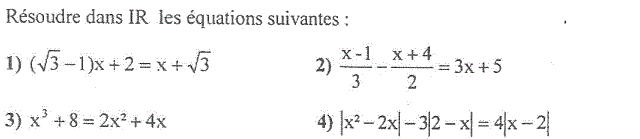
correction
Pour chacune des équations suivantes, on désigne par $S_\Bbb R$ son ensemble de solutions
1)
$$\begin{align*}
&(\sqrt{3}-1)x+2=x+\sqrt{3} \\
&\iff (\sqrt{3}-1)x-x=\sqrt{3}-2 \\
&\iff (\sqrt{3}-1-1)x=\sqrt{3}-2 \\
&\iff (\sqrt{3}-2)x=\sqrt{3}-2 \\
&\iff x=\frac{\sqrt{3}-2}{\sqrt{3}-2} \\
&\iff x=1
\end{align*}$$
Donc $\boxed{ S_\Bbb R=\left\{{1}\right\}}$
2)
$$\begin{align*}
&\frac{x-1}{3}-\frac{x+4}{2}=3x+5 \\
&\iff \frac{2(x-1)}{6}-\frac{3(x+4)}{6}=\frac{6(3x+5)}{6} \\
&\iff 2(x-1)-3(x+4)=6(3x+5) \\
&\iff 2x-2-3x-12=18x+30 \\
&\iff 2x-3x-18x=30+2+12 \\
&\iff -19x=44 \\
&\iff x=-\frac{44}{19}
\end{align*}$$
Donc $\boxed{S_\Bbb R=\left\{{-\frac{44}{19}}\right\}}$
3)
$$\begin{align*}
&x^3+8=2x^2+4x \\
&\iff x^3+2^3=2x(x+2) \\
&\iff (x+2)(x^2-2x+4)=2x(x+2) \\
&\iff (x+2)(x^2-2x+4)-2x(x+2)=0 \\
&\iff (x+2)(x^2-2x+4-2x)=0 \\
&\iff (x+2)(x^2-4x+4)=0 \\
&\iff (x+2)(x^2-2\times x\times 2+2^2)=0 \\
&\iff (x+2)(x-2)^2=0 \\
&\iff x+2=0 \; ou \; x-2=0 \\
&\iff x=-2 \; ou \; x=2
\end{align*}$$
Donc $\boxed{S_\Bbb R=\left\{{-2,2}\right\}}$
4)
$$\begin{align*}
&\left|{x^2-2x}\right|-3\left|{2-x}\right|=4\left|{x-2}\right| \\
&\iff |x(x-2)|-3|-(x-2)|=4|x-2| \\
&\iff |x(x-2)|-3|x-2|-4|x-2|=0 \\
&\iff |x(x-2)|-7|x-2|=0 \\
&\iff |x||x-2|-7|x-2|=0 \\
&\iff |x-2|(|x|-7)=0 \\
&\iff |x-2|=0\; ou \;|x|-7=0 \\
&\iff x=2 \; ou \;x=-7 \; ou \;x=7
\end{align*}$$
Donc $\boxed{S_\Bbb R=\left\{{-7,2,7}\right\}} $