Généralités sur les fonctions : Exercice 3 3eme année secondaire
Généralités sur les fonctionsProduit scalaire dans le planLimites et continuitéAngles orientésDérivabilité
33 exercices
Exercice 3 --- (id : 7)
correction
1
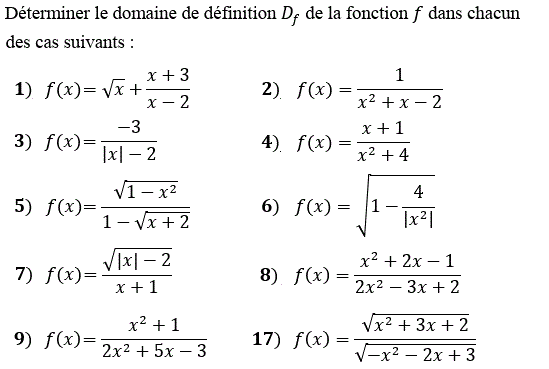
$f(x)=\sqrt x+\frac {x+3}{x-2}$
$f(x)$ existe $\Leftrightarrow x\geqslant 0\ et \ x-2\neq 0$ $\Leftrightarrow x\in \left[{0,+\infty}\right[╲\left\{{2}\right\}$ $\Longrightarrow \boxed{D_f=\left[{0,+\infty}\right[╲\left\{{2}\right\}}$
$f(x)$ existe $\Leftrightarrow x\geqslant 0\ et \ x-2\neq 0$ $\Leftrightarrow x\in \left[{0,+\infty}\right[╲\left\{{2}\right\}$ $\Longrightarrow \boxed{D_f=\left[{0,+\infty}\right[╲\left\{{2}\right\}}$
2
$f(x)=\frac {1}{x^2+x-2}$
$f(x)$ existe $\Leftrightarrow x^2+x-2\neq 0$ $\Leftrightarrow x\neq 1$ et $x\neq -2$ $ \Leftrightarrow x \in \Bbb R╲\left\{{1,-2}\right\} $ $\Longrightarrow \boxed{D_f=\Bbb R╲\left\{{1,-2}\right\} }$
$f(x)$ existe $\Leftrightarrow x^2+x-2\neq 0$ $\Leftrightarrow x\neq 1$ et $x\neq -2$ $ \Leftrightarrow x \in \Bbb R╲\left\{{1,-2}\right\} $ $\Longrightarrow \boxed{D_f=\Bbb R╲\left\{{1,-2}\right\} }$
3
$f(x)=\frac{-3}{\left|{x}\right|-2}$
$f(x)$ existe $\Leftrightarrow \left|{x}\right|-2\neq 0 \Leftrightarrow \left|{x}\right|\neq 2\Leftrightarrow x\neq-2\; et \; x\neq 2$ $\Longrightarrow \boxed{D_f=\Bbb R╲\left\{{-2,2}\right\} }$
$f(x)$ existe $\Leftrightarrow \left|{x}\right|-2\neq 0 \Leftrightarrow \left|{x}\right|\neq 2\Leftrightarrow x\neq-2\; et \; x\neq 2$ $\Longrightarrow \boxed{D_f=\Bbb R╲\left\{{-2,2}\right\} }$
4
$ f(x)=\frac{x+1}{x^2+4}$
$\forall x \in \Bbb R \; x^2+4\geqslant 4 \; donc \;non \;nul$ $\Longrightarrow \boxed{D_f=\Bbb R}$
$\forall x \in \Bbb R \; x^2+4\geqslant 4 \; donc \;non \;nul$ $\Longrightarrow \boxed{D_f=\Bbb R}$
5
$f(x)=\frac{\sqrt{1-x^2}}{1-\sqrt{x+2}}$
$f(x)\; existe\; \Leftrightarrow $ $1-x^2 \geqslant 0, x+2 \geqslant 0\;$ $et\; 1-\sqrt{x+2}\neq 0$
ou encore $x \in \left[{-1,1}\right]$ et $1-\sqrt{x+2}\neq 0$
Or $1-\sqrt{x+2}= 0$ $\Leftrightarrow 1=\sqrt{x+2}$ $\Leftrightarrow x+2=1 \;et\; x\geqslant -2$ ou encore $x=-1$
Alors $ \boxed{D_f=\left]{-1,1}\right]} $
$f(x)\; existe\; \Leftrightarrow $ $1-x^2 \geqslant 0, x+2 \geqslant 0\;$ $et\; 1-\sqrt{x+2}\neq 0$
ou encore $x \in \left[{-1,1}\right]$ et $1-\sqrt{x+2}\neq 0$
Or $1-\sqrt{x+2}= 0$ $\Leftrightarrow 1=\sqrt{x+2}$ $\Leftrightarrow x+2=1 \;et\; x\geqslant -2$ ou encore $x=-1$
Alors $ \boxed{D_f=\left]{-1,1}\right]} $
6
$ f(x)=\sqrt{1-\frac{4}{x^2}}$
$f(x)\; existe \Leftrightarrow $ $x\neq 0\; et 1-\frac{4}{x^2}\geqslant 0$ $\Leftrightarrow x\neq 0\;et \frac{4}{x^2}\leqslant 1$ $\Leftrightarrow x\neq 0\;et\; x^2-4\geqslant0$ $\Leftrightarrow x\in \left]{-\infty,-2}\right]\cup\left[{2,+\infty}\right[$ $\Longrightarrow \boxed{D_f= \left]{-\infty,-2}\right]\cup\left[{2,+\infty}\right[} $
$f(x)\; existe \Leftrightarrow $ $x\neq 0\; et 1-\frac{4}{x^2}\geqslant 0$ $\Leftrightarrow x\neq 0\;et \frac{4}{x^2}\leqslant 1$ $\Leftrightarrow x\neq 0\;et\; x^2-4\geqslant0$ $\Leftrightarrow x\in \left]{-\infty,-2}\right]\cup\left[{2,+\infty}\right[$ $\Longrightarrow \boxed{D_f= \left]{-\infty,-2}\right]\cup\left[{2,+\infty}\right[} $